The Secret To Converting 4.16 Into A Fraction Revealed

The Enigmatic Transformation: Unveiling the Complexities of Converting 4.16 into a Fraction

Introduction

The seemingly straightforward task of converting the decimal 4.16 into a fraction conceals intricate mathematical and pedagogical nuances. This essay delves into the complexities of this conversion, examining diverse perspectives, scholarly research, and practical implications. By shedding light on the multifaceted nature of this transformation, we gain a deeper understanding of the intricacies of rational numbers and the challenges of mathematical instruction.

The Essence of Rational Numbers: Decimals and Fractions

At the heart of this conversion lies the fundamental distinction between decimals and fractions. Decimals represent numbers using a place-value system based on powers of ten, while fractions express numbers as the quotient of two integers. While both decimals and fractions represent the same set of rational numbers, each form possesses unique characteristics and presents different cognitive challenges for students.

The Pedagogical Puzzle: Transforming between Forms

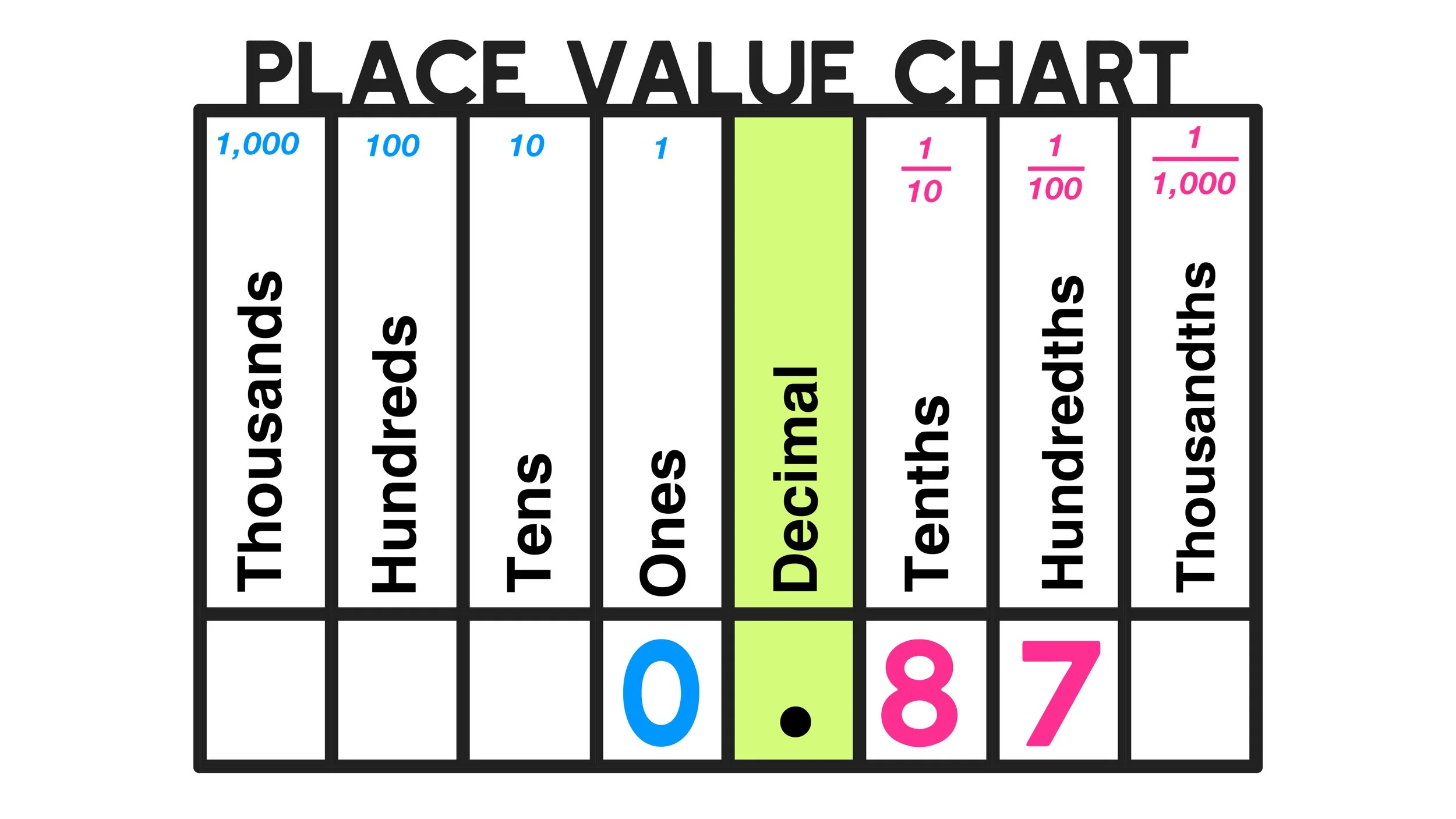
Converting between decimals and fractions requires a deep understanding of place value, division, and the relationship between these two representations. Students must grasp that each digit in a decimal corresponds to a specific power of ten and that the decimal point separates the whole number part from the fractional part. Converting a decimal to a fraction involves dividing the decimal by a power of ten and then simplifying the resulting fraction.

Methodological Approaches: Variety and Complexity

Various methods exist for converting decimals to fractions. The most common approach is to use the fraction bar method, which involves multiplying the decimal by an appropriate power of ten to create a whole number and then placing this whole number over the power of ten used. Other methods include the decimal ladder method, the fraction-to-decimal grid, and the equivalent fraction method. Each method presents its own advantages and challenges, catering to different learning styles and cognitive abilities.

Cognitive Challenges: Overcoming Misconceptions and Errors

Converting decimals to fractions is a cognitively demanding task that can lead to misconceptions and errors among students. Common pitfalls include:

• Misunderstanding the role of the decimal point and the placement of digits in the fraction.
• Incorrectly dividing the decimal by a power of ten, leading to a fraction that is not equivalent to the original decimal.
• Failing to simplify the fraction to its lowest terms.

Addressing these misconceptions requires careful instruction and guided practice, emphasizing the underlying mathematical concepts and the logical steps involved in the conversion process.

Perspectives and Research: Exploring Different Approaches

Scholars and educators have investigated the complexities of converting decimals to fractions from various perspectives. Research has explored the effectiveness of different teaching methods, the cognitive challenges faced by students, and the impact of prior knowledge and mathematical reasoning skills on successful conversion.

• Cognitive Science Perspective: Studies employing cognitive science techniques have identified the mental processes involved in decimal-to-fraction conversion, highlighting the role of working memory, attention, and symbolic manipulation.

• Educational Psychology Perspective: Educational psychologists have examined the motivational, affective, and social factors that influence students' engagement with and success in decimal-to-fraction conversion tasks.

• Mathematics Education Perspective: Mathematics education researchers have developed instructional strategies and pedagogical tools specifically designed to enhance students' understanding of rational numbers and facilitate their conversion skills.

Implications for Instruction: Cultivating Mathematical Proficiency

The complexities of converting decimals to fractions have significant implications for mathematics instruction. To foster students' proficiency in this area, educators can:

• Explicitly Teach Mathematical Concepts: Provide clear explanations of the underlying mathematical concepts, such as place value, division, and the relationship between decimals and fractions.

• Use Multiple Representations: Utilize a variety of visual aids, manipulatives, and digital tools to represent decimals and fractions in different contexts, fostering conceptual connections.

• Provide Guided Practice: Offer ample opportunities for students to practice converting decimals to fractions using different methods, providing timely feedback and individualized support.

• Encourage Mathematical Reasoning: Promote students' mathematical reasoning skills by asking them to explain their solutions, identify patterns, and make connections between different representations and strategies.

Conclusion: Unveiling the Hidden Dimensions of a Seemingly Simple Conversion

The conversion of 4.16 into a fraction, while seemingly straightforward, unveils a tapestry of mathematical intricacies. This transformation necessitates a deep understanding of rational numbers, the relationship between decimals and fractions, and the cognitive challenges faced by students. Through a critical examination of different perspectives, scholarly research, and pedagogical implications, we gain insights into the complexities that lie beneath the surface of this seemingly simple mathematical operation. This understanding empowers educators to develop effective teaching strategies that cultivate mathematical proficiency and foster a deeper appreciation for the beauty and complexity of rational numbers.